Effectively resolving mass events is one of the important tasks faced by Chinese governments at all levels in maintaining social harmony and stability. Mass events have some management characteristics such as strong suddenness caused by random interference factors, rapid evolution with the updated external environment, limited number of participants (from more than five people to thousands of people) and inconsistent interests of the protesters. The key step to successfully resolve events is that the protest group is divided into small groups and then some members accept the solution.
In this paper, in order to discover the resolution mechanism of mass evens, a stochastic evolutionary game model of mass event is proposed based on the Moran process in the limited population. Given the factor game of protesters that the protesters can choose to exit the protest strategy
W and continue to protest strategy
A, the probability that the number of protesters with the withdraw strategy increases by one unit is defined as
Pi,i+1=
ifi/
ifi+(
N-
i)
gi·(
N-
i)/
N, where the total number of protesters is
N, the number of protesters with the withdraw strategy is
i, the protesters' utility taken the withdraw strategy is,
fi=1-
β+
βπWi, and the protesters' utility taken the continuous protest is
gi=1-
β+
βπAi. The parameter
β denotes selection intensity,
β∈[0,1]. If
β→0,then it is weak selection, If
β=1, then it is strong selection, where the utility function is equal to the relative payoff.
Then, the impact of selection intensity on the evolutionary equilibrium of mass events is analyzed. If random elements dominate the evolution of events, i.e.
β→0, the strategy of withdraw protest
W will become a evolutionary equilibrium in the condition of
ρW=1/(1+
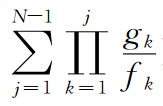
)>1/
N, where
ρW means the fixation probability of
W strategy. If the deterministic factors dominate the evolution of events, i.e.
β=1, the strategy of withdraw protest
W will become a evolutionary equilibrium in the condition of
h1>0,
hN-1>0, where h is the difference of utility function between withdraw protest strategy
W and continue protest strategy
A. The above results provide the necessary condition and resolution mechanism for mass events. Lastly, the numerical analysis is taken based on the land expropriation compensation incident in Dongguan city.
The results show that the mass events are resolved successfully, when the interference of random factors is relatively small, the economic resources that are competed by governments and protesters is lower, the government compensation and the cost of conflict are higher, and the fixation probability that the protesters exit the protest is larger. The scale of the protesters has a threshold at which protests can be successfully defused. Comparing the weak selection process dominated by random factors with the strong selection process excluding random factors, the latter has a smaller critical scale of protesters.
In conclusion, a new theoretical explanation about the resolution mechanism of mass events is provided based on the stochastic evolutionary game theory. Moreover, an abundant management inspiration for local governments is provided to resolve mass events effectively. For example, the size of the protesters is an important factor in the successful resolution of the mass events. In the initial stage of the mass incident where the external random factors and the size of the group participating are small, the local government should increase the disposal intensity. The government adheres to the principle of resolving the rule of law channels and increases the punishment for illegal acts of beating, smashing and looting, which is conducive to the resolution of events and so on.


